Calculus is a branch of mathematics that deals with functions, limits, derivatives, and integrals. It is a fascinating subject that has many practical applications in science, engineering, economics, and finance.
What is Calculus?
Calculus is a type of advanced math that involves the study of how things change. It is broken down into two areas: differential calculus and integral calculus. Differential calculus deals with the study of how things change over time, while integral calculus examines the accumulation of factors over time.
Calculus is useful in many fields because it enables the prediction of how things will change. Engineers use calculus to design structures and machines, while economists use it to analyze markets and trends. Physicists use calculus to study the behavior of energy and matter, while astronomers use it to study the movement of celestial bodies. Calculus is also used in medicine, computer science, and many other fields.
Limit Definition of the Derivative
The derivative is a mathematical function that describes the rate at which one variable changes in relation to another. It is an essential concept in calculus and is used extensively in physics and engineering. The limit definition of the derivative is a method for calculating the derivative of a function at a particular point.
Problem 5
In problem 5, we are given a function f(x) = x^2 + 1 and asked to find the derivative f'(x) at the point x = 2. Using the limit definition of the derivative, we can calculate f'(2) by taking the limit as h approaches 0 of [f(2+h) - f(2)]/h.
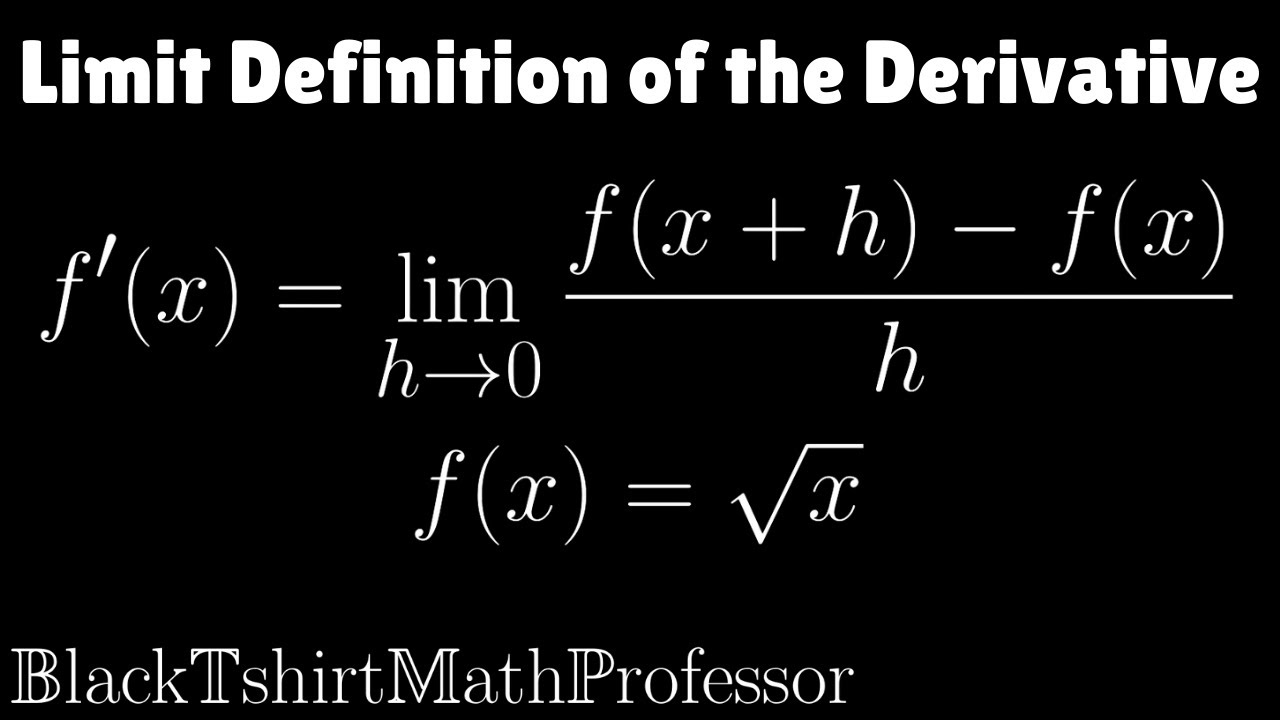
Substituting the values from the problem into the equation gives us [f(2+h) - f(2)]/h = [(2+h)^2 + 1 - 5]/h. Simplifying this expression yields (4h + h^2)/h, which can be further reduced to 4 + h using algebraic manipulation. Taking the limit as h approaches 0, we find that the derivative of f(x) at x = 2 is equal to 4.
Conclusion
Calculus is a fascinating subject that has numerous practical applications in many fields, including science, engineering, economics, finance, and medicine. The limit definition of the derivative is an essential concept in calculus and is used extensively in physics and engineering. By understanding these concepts, we can gain a deeper appreciation for the world around us and the ways in which it operates.
If you are searching about Calculus Meaning - YouTube you've came to the right page. We have 6 Pictures about Calculus Meaning - YouTube like What Is Calculus by tutorcircle team - Issuu, Calculus Meaning - YouTube and also Calculus word cloud collage, education concept background. | CanStock. Here you go:
Calculus Meaning - YouTube
www.youtube.com calculus zealous meaning
What Is Calculus? Definition And Practical Applications
:max_bytes(150000):strip_icc()/calculus-on-blackboard-79338340-5be4695946e0fb0026d6856f.jpg)
www.thoughtco.com calculus getty
Calculus Books For Machine Learning – AiProBlog.Com
www.aiproblog.com calculus
Calculus Word Cloud Collage, Education Concept Background. | CanStock
www.canstockphoto.com calculus
What Is Calculus By Tutorcircle Team - Issuu
issuu.com calculus
Limit Definition Of The Derivative F'(x) Problem 5 (Calculus 1) - YouTube
www.youtube.com calculus derivative
What is calculus? definition and practical applications. Calculus meaning. Calculus derivative
No comments:
Post a Comment