The concept of integral calculus might seem like rocket science to some, but trust me, it's not as complicated as it sounds. Simply put, integral calculus is a branch of calculus that deals with the integration of functions, aka finding the area under a curve. To understand integral calculus, we first need to understand what a function is. In math, a function is basically a set of instructions that tells you how to take one number and turn it into another number. For example, the function f(x) = 2x tells us that if we put in 3 for x, we'll get 6 as the output. Now, imagine that we have some sort of curve on a graph that represents this function. The area under this curve between two points, say a and b, is what we call the definite integral of the function from a to b. So how do we actually find this area? Well, we use something called the Riemann Sum, which is a fancy way of saying that we divide our area into smaller and smaller rectangles, compute the area of each one, and then add them all up. But here's the thing - a Riemann Sum is not the most efficient way to calculate integrals. Instead, we use something called the Fundamental Theorem of Calculus, which tells us that the definite integral of a function can be found by evaluating the antiderivative of that function at the upper and lower limits of integration. Wait, what's an antiderivative? Good question. If a function tells us how to go from one number to another, the antiderivative is like the opposite of that. It tells us what function we need to differentiate in order to get the original function back. So if the function f(x) = 2x, the antiderivative would be F(x) = x^2 + C (where C is a constant). Now, let's put all of this into action. Imagine we want to find the area under the curve of the function f(x) = x^2 between x = 0 and x = 2. Using the Fundamental Theorem of Calculus, we can find the antiderivative of f(x), which is F(x) = (1/3)x^3. Then we simply evaluate this antiderivative at x = 2 and x = 0, and subtract the two values to get our answer: (1/3)(2^3) - (1/3)(0^3) = 8/3. Voila! We've successfully found the area under the curve using integral calculus. Of course, there are many more complex functions out there that require more advanced techniques, but the basic principles are the same. So don't let the complexity of integral calculus scare you - with a bit of practice, you'll be integrating like a pro in no time.
If you are looking for Integral Calculus - YouTube you've visit to the right place. We have 6 Images about Integral Calculus - YouTube like INTEGRAL CALCULUS (PART-2) - YouTube, INTEGRAL CALCULUS - TRAJECTORY ONLINE and also Math Cheat Sheets - Математикийн Шипи | I CAME EARTH FOR EXP. Here it is:
Integral Calculus - YouTube
 www.youtube.com
www.youtube.com calculus
INTEGRAL CALCULUS - TRAJECTORY ONLINE
 trajectoryonline.com
trajectoryonline.com calculus samvedna
MATH 101 – Integral Calculus With Applications To Physical Sciences
 studylib.net
studylib.net Integral Calculus
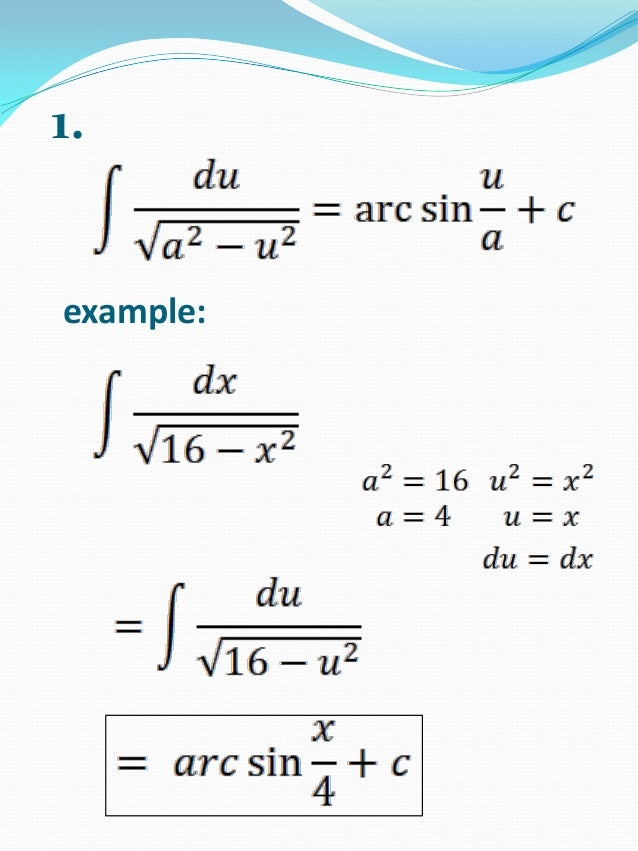 www.slideshare.net
www.slideshare.net integral calculus
Math Cheat Sheets - Математикийн Шипи | I CAME EARTH FOR EXP
 www.olexir.com
www.olexir.com cheat calculus sheet integral formula pdf integrals calc maths math printable exp trig
INTEGRAL CALCULUS (PART-2) - YouTube
 www.youtube.com
www.youtube.com calculus
Math 101 – integral calculus with applications to physical sciences. Integral calculus. Integral calculus (part-2)
 www.youtube.com
www.youtube.com  trajectoryonline.com
trajectoryonline.com  studylib.net
studylib.net  www.slideshare.net
www.slideshare.net  www.olexir.com
www.olexir.com  www.youtube.com
www.youtube.com 
No comments:
Post a Comment