Have you ever heard of the definite integral? It may sound like a complicated concept, but it's actually quite simple once you understand the basics. In essence, the definite integral is a way of measuring the area under a curve. This concept has many applications in math, science, and engineering, making it an important tool for anyone interested in these fields. To understand the definite integral, let's start by looking at a simple example. Imagine you have a straight line graph that begins at the point (0,0) and ends at (5,5). To find the area under this line, you would simply calculate the area of the rectangle that it forms. In this case, the length of the rectangle is 5 units and the height is also 5 units, so the area is 25 square units. But what if you have a more complex curve, like a parabola or a sin wave? In these cases, you can't simply calculate the area using basic geometry. This is where the definite integral comes in. Essentially, the process of finding the definite integral involves dividing the area under the curve into small, infinitely thin rectangles, calculating the area of each rectangle, and then adding all of the rectangles together. To write the formula for the definite integral, we use the following notation: ∫_(a to b) f(x) dx The integral symbol (which looks like an elongated "S") represents the process of finding the area under the curve. The "a" value represents the starting point of the curve, and the "b" value represents the ending point. The "f(x)" term represents the height of the curve at each point, and the "dx" term indicates that we're calculating the area with respect to the x-axis (as opposed to the y-axis). To illustrate this concept, let's look at an example. Imagine we have a curve defined by the equation y = x^2, and we want to find the area under this curve between the points x = 0 and x = 2. To do this, we can use the following formula: ∫_(0 to 2) x^2 dx To solve this integral, we need to evaluate the antiderivative of the equation x^2. In this case, the antiderivative is x^3/3. So the definite integral becomes: ∫_(0 to 2) x^2 dx = [x^3/3]_(0 to 2) = (2^3/3) - (0^3/3) = 8/3 This means that the area under the curve between x = 0 and x = 2 is approximately 2.67 square units. As you can see, the definite integral is a powerful tool for calculating the area under complex curves. It has many practical applications in fields like physics, engineering, and economics. So if you're interested in pursuing a career in any of these areas, it's essential to have a solid understanding of this fundamental concept.
If you are searching about The Definition of The Definite Integral | Definitions, Study, Continuity you've came to the right page. We have 6 Images about The Definition of The Definite Integral | Definitions, Study, Continuity like The Definite Integral, The Definition of The Definite Integral | Definitions, Study, Continuity and also Definite Integral - YouTube. Here it is:
The Definition Of The Definite Integral | Definitions, Study, Continuity
www.pinterest.com definite definitions
Definite Integral - YouTube
www.youtube.com definite integral integration examples calculus basic
PPT - Introduction To Integrals PowerPoint Presentation, Free Download
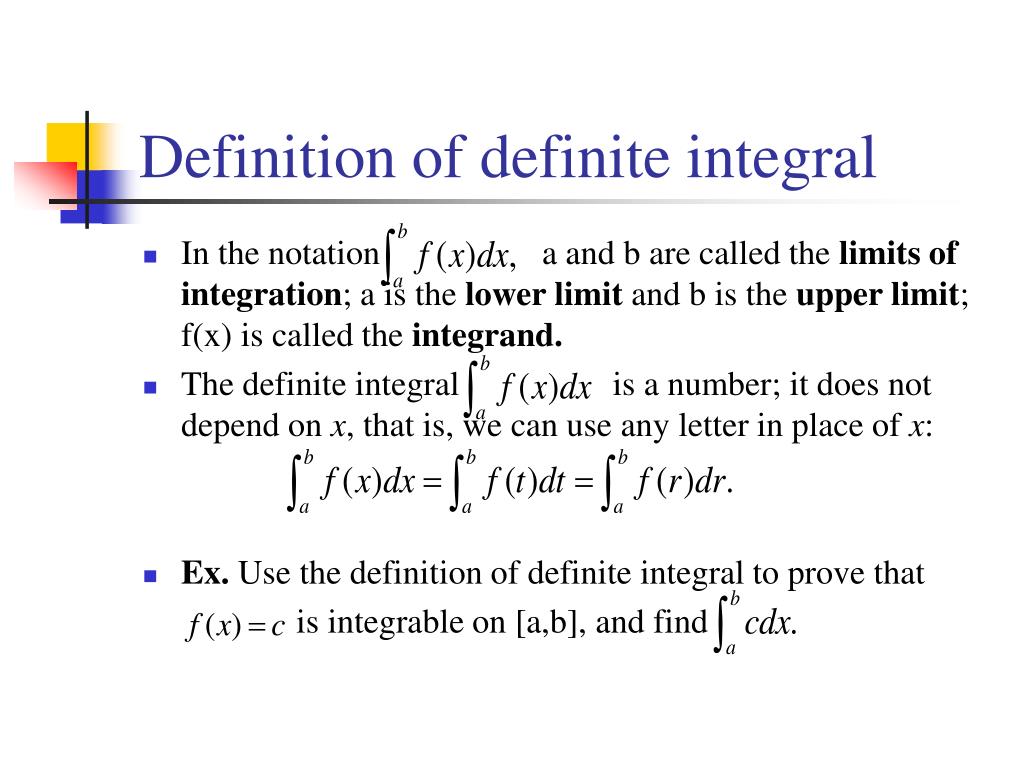www.slideserve.com definition definite integrals integral limit introduction limits lower
The Definite Integral
studylib.net integral definite definition closed doc
The Definite Integral | Teaching Resources
www.tes.com definite integral resources
Solved Evaluate Integral^2_0 (2x - X^3) Dx Using The | Chegg.com
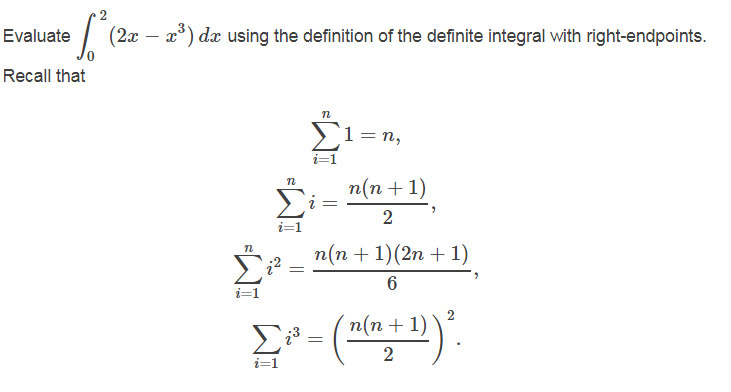www.chegg.com integral definite evaluate endpoints transcribed
Definite integral. Definite integral resources. The definition of the definite integral
No comments:
Post a Comment